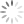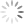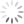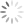Blog entry by Ariane Stern & Julie Kim

First Graders Explore Area and Perimeter

Earlier in the year, we introduced the concept of area—that you can measure how small or large a space is. After measuring various shapes with non-standard units (e.g. tiles, beans, paperclips), first graders developed an understanding that with bigger units like popsicle sticks, you’d need fewer of them to cover a certain area and that you would need many more small units like beans to fill that same area. Later in the  year, we began a unit on linear measurement. Again, we used manipulatives to measure the length of objects. This time, we focused  on ways to accurately measure length, such as figuring out which side is the length, and measuring by starting at the edge. After learning how to measure length accurately with one unit, we moved again to the idea of measuring the same object with different sized units. Once again, it takes less popsicle sticks to measure the length of a book, and more cubes, if you were measuring the same length.

 This year, to culminate our study on linear measurement, we challenged first graders to measure how long each Lower School classroom’s block area is. In small groups, first graders went to each classroom, calculated which side was the longest side and measured it with string. They brought their strings back to our classroom and proceeded to measure how long they were with popsicle sticks, tiles, and double unit wooden blocks. Afterwards, we collected each group’s data and compared the number of double unit blocks it took to measure the length of each string. We put the lengths in order to see which block area was the longest.

Along the way, we ran into some problems, just as mathematicians do in real life! What would we do if the double units were too long or too short for the end of the string? That led us into a conversation about fractions. We looked at halves, quarters, and thirds of rectangles and also looked at circles to deepen our understanding of fractions. This helped some groups to make more accurate measurements.

Then, Julie showed the class some pictures. Tasha’s block area was the longest, but it was very narrow and skinny. Diane’s block area was the shortest, but it was very wide. We wondered, just because a block area is the longest, does that mean it’s the biggest?

What a big question!

How would we figure that out? We knew what all the lengths of the block areas were, but how would we figure out how big they were? What were we even looking for? One student shouted, “The area! How were we going to figure out the area? We drew a rectangle on the board, showing how we knew the lengths. What else would we need to know to find the area? This was a puzzle. But calling upon what we had already learned about area, we realized that we needed to know the width of each block area. Wow!

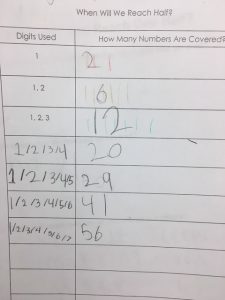
Each group then went back to their assigned classroom, this time measuring the width of each block area. When they came back, they measured their new strings again with double unit wooden blocks. Then they used graph paper to replicate their block area. Each square of the graph paper represented one double unit block. Then they counted all the squares to figure out the area. This was hard work! Many of the shapes they drew had over 100 squares! Each group had to count the squares in their shape multiple times to try and get the most accurate count.

At the end of the day, we did figure out which classroom had the largest block area.

It wasn’t the longest block area!